Workshops Organization
AHGT - Arithmetic & Homotopic Galois Theory 2023 workshop, RIMS-ENS PSL hybrid, 2023.
Organizer with P. Dèbes, Y. Hoshi and A. Mézard of the opening workshop of the CNRS France-Japan AHGT international reseach network between RIMS Kyoto, LPP Lille University and DMA ENS PSL -- see Programme and participants.
RIMS - Expanding Horizons of Inter-universal Teichmüller Theory, 2021.
I have been invited to join Professor S. Mochizuki (RIMS, Kyoto) I. Fesenko (Nottingham, UK), Y. Hoshi (RIMS, Kyoto) and Y. Taguchi (Titech, Tokyo) in the co-organization of the four workshops series of special RIMS year ``Expanding Horizons of Inter-universal Teichmüller Theory''.
Foundations and Perspectives of Anabelian Geometry. Review fundamental developments in anabelian geometry and report on recent developments - participants and programme (June 28 - July 2, 2021) [Notes].
Combinatorial Anabelian Geometry & Related Topics. Fundamental, introductory aspects of combinatorial anabelian geometry, developments related to the Grothendieck-Teichmüller group and the absolute Galois groups of number fields and mixed-characteristic local fields - participants and programme (July 6 - July 9, 2021) [Notes].
Invitation to Inter-universal Teichmüller Theory. Towards beginning learners of IUT, for further dissemination of principles and techniques of IUT - participants and programme (Aug. 31 - Sept. 3, 2021) [Notes].
Inter-universal Teichmüller theory Summit. For advanced learners of IUT, on recent progress and advances - participants and programme (Setp. 7 - Sept. 10, 2021) [Notes].
Oberwolfach - Homotopic and Geometric Galois Theory, 2021.
Organizer with P. Dèbes, H. Nakamura and J. Stix of a workshop on the recent developments in abelian & and anabelian Geometric Galois & Inverse Galois theories - see Report of the workshop (82 pages).
A fundamental idea in studying the absolute Galois group of a field is to make it act on geometric objects such as Galois covers, étale cohomology groups and fundamental groups. Striking advances have recently shed new light on the seminal topics of (a) Galois Covers, (b) Motivic Representations, and (c) Anabelian Geometry. Essential crossbridging principles connect these advances: homotopic methods, higher stacks, Tannakian symmetries. Based on the recent results and their promising connections, and on the 2018 MFO mini-workshop in a similar spirit, this workshop aims to crystallize these innovative approaches and to strengthen fruitful desire paths in homotopic and geometric Galois theory.
Oberwolfach - Arithmetic Geometry and Symmetries around Galois and Fundamental Groups, 2018.
Organizer with P. Dèbes and M. Fried of a mini-workshop on the recent developments in abelian & and anabelian Geometric Galois & Inverse Galois theories - see Report of the workshop (60 pages).
The geometric study of the absolute Galois group of the rational numbers has been a highly active research topic since the first milestones: Hilbert’s Irreducibility Theorem, Noether’s program, Riemann’s Existence Theorem. It gained special interest in the last decades with Grothendieck’s “Esquisse d’un programme”, his “Letter to Faltings” and Fried’s introduction of Hurwitz spaces. It grew on and thrived on a wide range of areas, e.g. formal algebraic geometry, Diophantine geometry, group theory. The recent years have seen the development and integration in algebraic geometry and Galois theory of new advanced techniques from algebraic stacks, l-adic representations and homotopy theories. It was the goal of this mini-workshop, to bring together an international panel of young and senior experts to draw bridges towards these fields of research and to incorporate new methods, techniques and structures in the development of geometric Galois theory.
Seminars Organization
Promenade in Inter-Universal Teichmüller Theory, RIMS Kyoto - Lille Seminar, 2020-21.
Mochizuki's Inter-Universal Teichmüller theory provides a new geometry of Diophantine properties of the moduli stack of elliptic curves based on a deconstruction-reconstruction process -- or Fukugen 復元 -- of absolute mono-anabelian geometry.
The goal of this seminar between Painlevé-Lille (France) and RIMS-Kyoto (Japan) — September 2020 - April 2021 — is for generic arithmetic-geometers to gain a general understanding of results, insights and techniques of IUT.
Zoom on-line seminar; participants from Kyoto, Tokyo, Osaka (JP); Lille (FR); Nottingham (UK).
See [Webpage of the Seminar] :: [Programme and references - PDF]
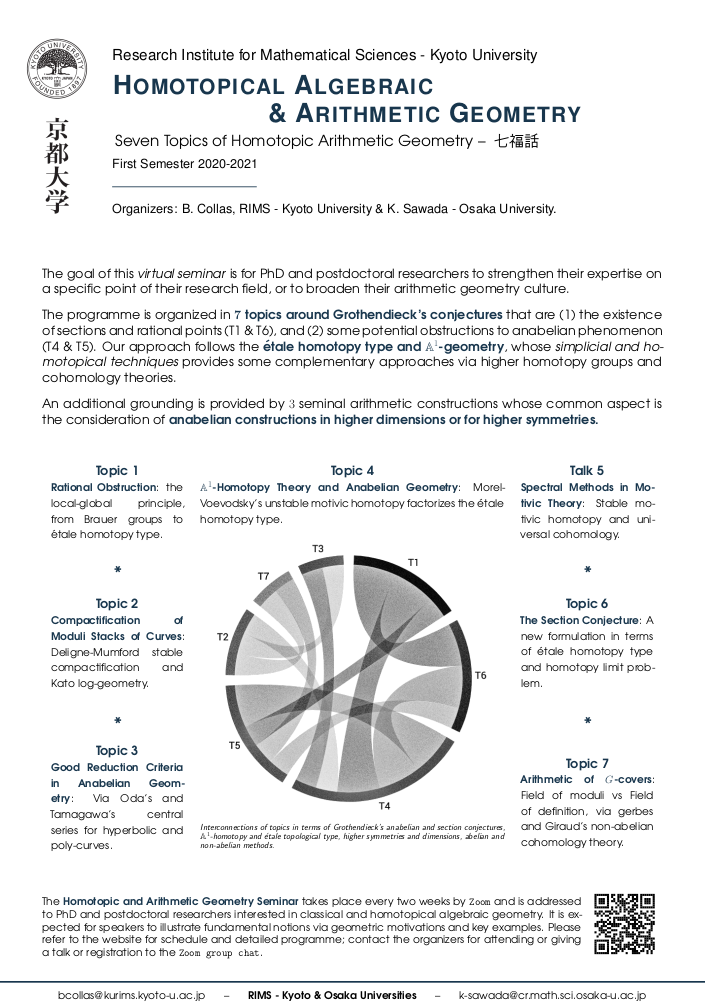
Homotopical Arithmetic Geometry Seminar - 7 Topics - 七福話, RIMS Kyoto Seminar, 2020.
We investigate 7 topics around Grothendieck's conjectures that are (1) the existence of sections and rational points (T1 & T6), and (2) some potential obstructions to anabelian phenomenon (T4 & T5). Our approach follows the étale homotopy type and A1-geometry, whose simplicial and homotopical techniques provides some complementary approaches via higher homotopy groups and cohomology theories.
An additional grounding is provided by 3 seminal arithmetic constructions whose common aspect is the consideration of anabelian constructions in higher dimensions or for higher symmetries.
Zoom on-line seminar; in English & Japanese; participants from Kyoto, Tokyo, Osaka (JP); Lille, Grenoble (FR); Nottingham (UK).
See [Webpage of the Seminar] :: [Programme and references - PDF]
Homotopical Anabelian Geometry, RIMS Kyoto Seminar, 2019.
We present the recent anabelian results that ensue the introduction of Artin-Mazur and Friedlander homotopical étale context in arithmetic-geometry by A. Schmidt and J. Stix -- an étale homotopy type reformulation of Mochizuki’s Theorem A, a higher dimensional anabelian result: every point of smooth variety over number fields admits an anabelian Artin neighbourhood (see also Hoshi’s generalization from number to generalized sub-p-adic field for polycurves.) A special attention is given on how classical and homotopical anabelian geometry interact together.
In a broader perspective, homotopical geometry provides new insights in arithmetic geometry for example in terms of stacks and motivic homotopy theory.
In English & Japanese; participants from Kyoto and Osaka (JP).
See [Webpage of the Seminar] :: [Programme and references - PDF]
Weekly Seminars Organization
Arithmetic-Geometry Seminar, LIV Bayreuth, Germany.
From 2014 to 2017, I was the organizer of the Arithmetic-Geometry seminar which is a joint weekly seminar between Prof. Dettweiler's and Prof. Stoll's chairs.
Leray Seminar, WWU Muenster, Germany.
From 2012 to 2014, I was the organizer of the Leray Algebraic Topology seminar for Michael Weiss. I also organized some specials sessions around the topic of Operads.